How to Calculate Rectangle Area Moment of Inertia

Calcululating the area moment of inertia for a rectangle is a fundamental concept in engineering and physics, essential for analyzing structural integrity and material behavior. Whether you’re a student, engineer, or enthusiast, understanding this calculation is crucial for various applications. In this guide, we’ll walk you through the step-by-step process, providing clear instructions and practical tips to master this essential skill. (rectangle area moment of inertia, moment of inertia calculation, structural engineering)
Understanding the Basics of Area Moment of Inertia
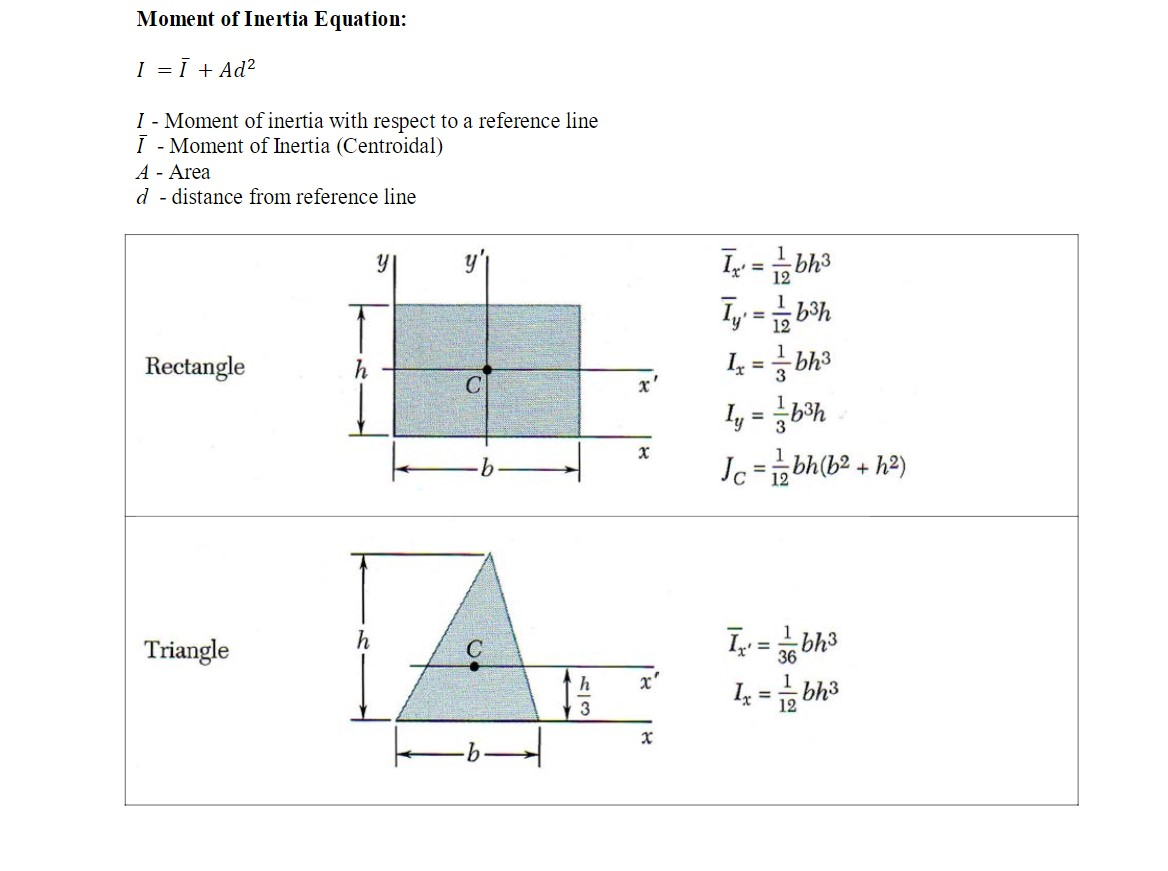
The area moment of inertia, often denoted as ( I ), measures an object’s resistance to bending or deformation. For a rectangle, it’s calculated about a specific axis, typically the x-axis or y-axis. This value is vital in designing beams, columns, and other structural elements. (moment of inertia, structural analysis, beam design)
Key Formula for Rectangle Area Moment of Inertia
The formula for the area moment of inertia of a rectangle is:
[ I = \frac{1}{12} b h^3 ]
Where: - ( b ) = width of the rectangle - ( h ) = height of the rectangle
This formula applies when the axis passes through the centroid of the rectangle. (rectangle moment of inertia formula, centroid calculation)
Step-by-Step Guide to Calculate Rectangle Area Moment of Inertia

Step 1: Identify the Rectangle Dimensions
First, measure or determine the width (( b )) and height (( h )) of the rectangle. Accurate dimensions are critical for precise calculations. (rectangle dimensions, measurement techniques)
Step 2: Apply the Formula
Substitute the values of ( b ) and ( h ) into the formula:
[ I = \frac{1}{12} b h^3 ]
Ensure you follow the order of operations (PEMDAS/BODMAS) for accurate results. (mathematical operations, calculation accuracy)
Step 3: Calculate the Result
Perform the calculation to find the area moment of inertia. For example, if ( b = 2 ) meters and ( h = 4 ) meters:
[ I = \frac{1}{12} \times 2 \times 4^3 = \frac{1}{12} \times 2 \times 64 = \frac{128}{12} \approx 10.67 \, \text{m}^4 ]
📝 Note: Always double-check your units to ensure consistency throughout the calculation.
Practical Applications of Rectangle Area Moment of Inertia

Understanding how to calculate the area moment of inertia is essential in: - Structural Engineering: Designing beams and columns to withstand bending stresses. (structural design, stress analysis) - Mechanical Engineering: Analyzing the deflection of rectangular components under load. (mechanical analysis, load testing) - Material Science: Studying the behavior of materials under different conditions. (material properties, deformation analysis)
Checklist for Calculating Rectangle Area Moment of Inertia
- [ ] Measure the width (( b )) and height (( h )) of the rectangle.
- [ ] Apply the formula ( I = \frac{1}{12} b h^3 ).
- [ ] Ensure consistent units throughout the calculation.
- [ ] Double-check the result for accuracy.
By following these steps, you’ll be able to calculate the area moment of inertia for any rectangle with confidence. (rectangle calculations, engineering fundamentals)
What is the area moment of inertia?
+The area moment of inertia is a property of a shape that describes its resistance to bending or deformation, often used in structural and mechanical engineering.
Why is the area moment of inertia important?
+It helps engineers analyze how structures or components will behave under stress, ensuring safety and efficiency in design.
Can the formula be used for shapes other than rectangles?
+No, this specific formula is for rectangles. Other shapes require different formulas based on their geometry.
Mastering the calculation of the rectangle area moment of inertia is a valuable skill for anyone involved in engineering or physics. By following the steps outlined in this guide, you’ll be well-equipped to tackle real-world problems with confidence. Remember, accuracy in measurements and calculations is key to successful applications. (engineering calculations, physics fundamentals, structural integrity)



