Mastering Cubic Factorization: A Step-by-Step Guide

Mastering cubic factorization is a crucial skill in algebra, enabling you to simplify complex polynomial expressions efficiently. Whether you're a student preparing for exams or a professional tackling real-world problems, understanding this technique is invaluable. In this guide, we'll walk you through the process step-by-step, ensuring you grasp the concepts and apply them confidently. (cubic factorization, polynomial simplification, algebra techniques)
Understanding Cubic Factorization Basics

Before diving into the steps, let’s establish a solid foundation. Cubic factorization involves breaking down a cubic polynomial into its constituent factors, often using methods like grouping or synthetic division. This process is essential for solving equations, graphing functions, and analyzing mathematical models. (cubic polynomials, factorization methods, mathematical modeling)
Step 1: Identify the Cubic Polynomial
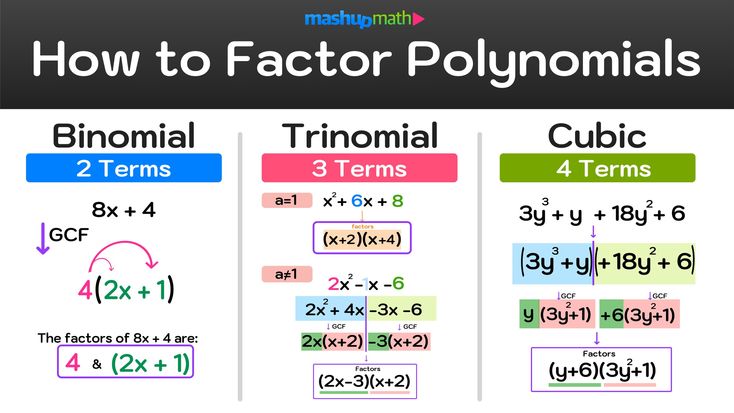
Begin by recognizing the cubic polynomial you need to factorize. A cubic polynomial has the general form: ax³ + bx² + cx + d, where a ≠ 0. Ensure you understand the coefficients and the degree of the polynomial. (polynomial identification, coefficients, degree of polynomial)
Step 2: Factor by Grouping

One effective method is factoring by grouping. This involves dividing the polynomial into pairs and factoring out common terms. For example, given x³ + 5x² + 3x + 15, group as (x³ + 5x²) + (3x + 15), then factor out common terms: x²(x + 5) + 3(x + 5). Notice the common factor (x + 5), leading to the factored form: (x + 5)(x² + 3). (factoring by grouping, common terms, polynomial pairs)
📌 Note: Factoring by grouping works best when the polynomial has four terms and can be split into two pairs with common factors.
Step 3: Use Synthetic Division
For more complex polynomials, synthetic division is a powerful tool. This method involves dividing the polynomial by a linear factor, often identified using the Rational Root Theorem. For instance, to factor x³ - 6x² + 11x - 6, test possible roots like x = 1 or x = 2. Upon finding a root, use synthetic division to simplify the polynomial. (synthetic division, Rational Root Theorem, polynomial roots)
| Step | Bring Down | Multiply | Add |
|---|---|---|---|
| 1 | 1 | 1 × 2 = 2 | 2 - 6 = -4 |
| 2 | -4 | -4 × 2 = -8 | -8 + 11 = 3 |
| 3 | 3 | 3 × 2 = 6 | 6 - 6 = 0 |

📌 Note: Synthetic division is efficient for polynomials with known or easily testable roots.
Step 4: Apply the Remainder Theorem

The Remainder Theorem states that if a polynomial f(x) is divided by (x - c), the remainder is f©. Use this theorem to verify your factorization. For example, if f(x) = x³ - 6x² + 11x - 6 and you’ve factored it as (x - 1)(x - 2)(x - 3), check f(1), f(2), and f(3) to ensure they equal zero. (Remainder Theorem, polynomial verification, factor confirmation)
Cubic Factorization Checklist
- Identify the cubic polynomial and its coefficients.
- Attempt factoring by grouping for four-term polynomials.
- Use synthetic division for complex polynomials with known roots.
- Apply the Remainder Theorem to verify your factorization.
Mastering cubic factorization opens up new possibilities in algebra and beyond. By following these steps—identifying polynomials, factoring by grouping, using synthetic division, and applying the Remainder Theorem—you'll tackle even the most challenging problems with confidence. Practice regularly, and soon, cubic factorization will become second nature. (algebra mastery, problem-solving skills, mathematical confidence)
What is cubic factorization?
+Cubic factorization is the process of breaking down a cubic polynomial into its constituent factors, simplifying complex expressions for easier analysis and problem-solving.
When should I use synthetic division?
+Use synthetic division when factoring complex polynomials with known or easily testable roots, as it provides an efficient way to simplify the expression.
How do I verify my factorization?
+Apply the Remainder Theorem by checking if the polynomial equals zero at the roots of the factored form. This confirms the accuracy of your factorization.



