Understanding the Concept of Inverse in Addition
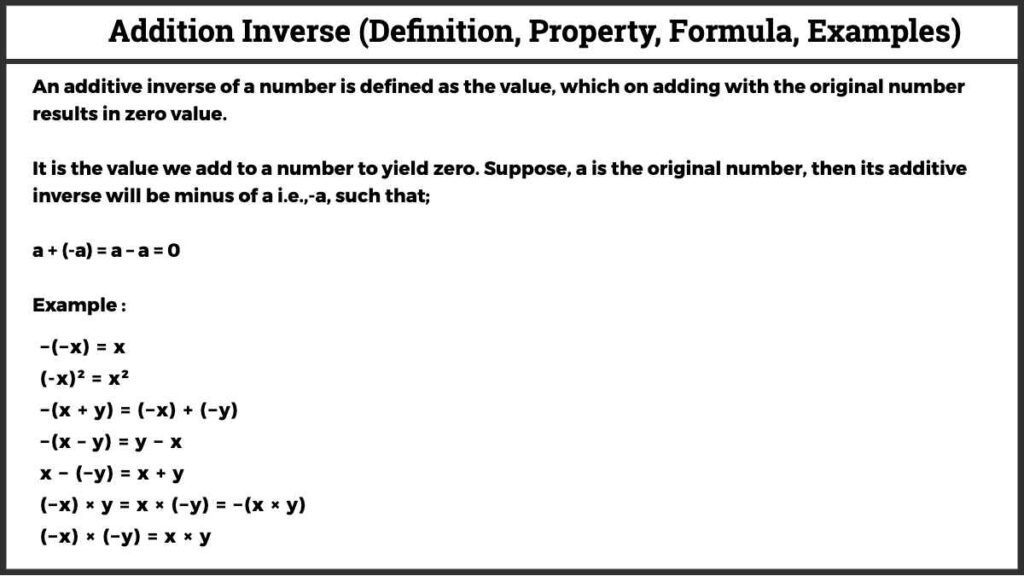
Understanding the concept of inverse in addition is essential for anyone looking to strengthen their mathematical foundation. In simple terms, the inverse in addition refers to the operation that, when applied to a number, results in the additive identity, which is zero. This concept is crucial in various mathematical applications, from basic arithmetic to advanced algebra. By grasping this idea, you can solve equations more efficiently and understand the relationships between numbers better. Whether you’re a student, educator, or simply curious about math, this guide will break down the concept into easy-to-understand segments.
What is the Inverse in Addition?

The inverse in addition is essentially the opposite of a number, also known as the additive inverse. For any given number, its additive inverse is the value that, when added to the original number, equals zero. For example, the additive inverse of 5 is -5, because 5 + (-5) = 0. This concept is fundamental in mathematics and serves as the basis for understanding more complex operations like subtraction and solving equations.
💡 Note: The additive inverse is unique for every number and always results in zero when added to the original number.
How to Find the Additive Inverse

Finding the additive inverse is straightforward. Follow these steps:
- Identify the Number: Start by identifying the number for which you want to find the additive inverse.
- Change the Sign: If the number is positive, change it to negative. If it’s negative, change it to positive.
- Verify the Result: Add the original number and its inverse to ensure the sum is zero.
| Number | Additive Inverse | Sum |
|---|---|---|
| 7 | -7 | 0 |
| -3 | 3 | 0 |

Practical Applications of Additive Inverse
The concept of additive inverse is not just theoretical; it has practical applications in real-world scenarios. For instance:
- Finance: Balancing budgets or calculating debts.
- Physics: Resolving forces in opposite directions.
- Computer Science: Binary operations and data manipulation.
Additive Inverse in Equation Solving

Understanding the additive inverse is key to solving linear equations. For example, to solve for x in the equation x + 5 = 0, you would use the additive inverse of 5, which is -5. Thus, x = -5. This method simplifies equation solving and is a foundational skill in algebra.
Checklist for Mastering Additive Inverse
- Identify: Recognize the number for which you need the additive inverse.
- Change: Flip the sign of the number.
- Verify: Ensure the sum of the number and its inverse equals zero.
- Apply: Use the concept in solving equations and real-world problems.
Mastering the concept of inverse in addition opens doors to advanced mathematical understanding and practical problem-solving. By following the steps and applying the knowledge, you’ll find it easier to tackle more complex mathematical challenges. additive inverse, equation solving, mathematical foundation
What is the additive inverse of a positive number?
+The additive inverse of a positive number is its negative counterpart. For example, the additive inverse of 8 is -8.
Can zero have an additive inverse?
+Yes, the additive inverse of zero is zero itself, because 0 + 0 = 0.
How is the additive inverse used in subtraction?
+Subtraction can be thought of as adding the additive inverse. For example, 5 - 3 is the same as 5 + (-3).



