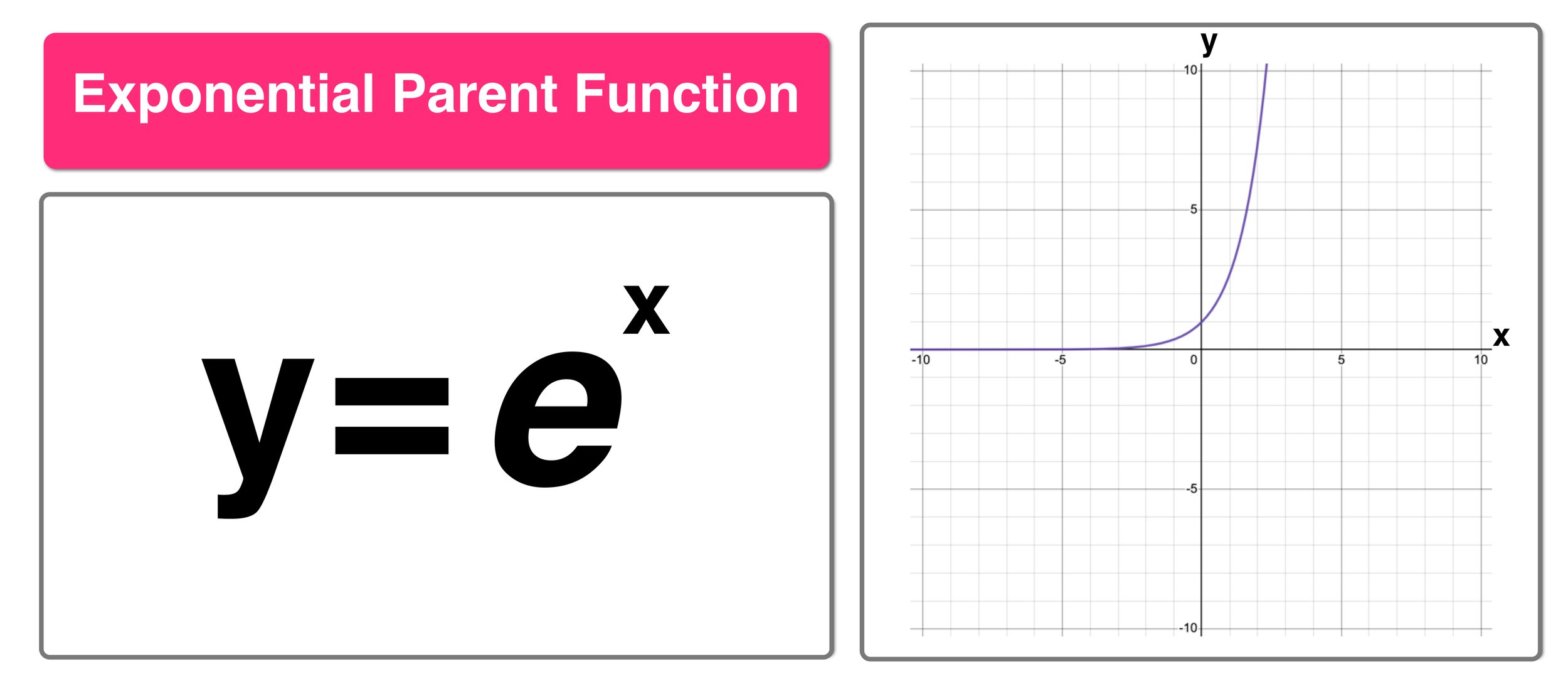
Understanding the Parent Function of Exponential Decay
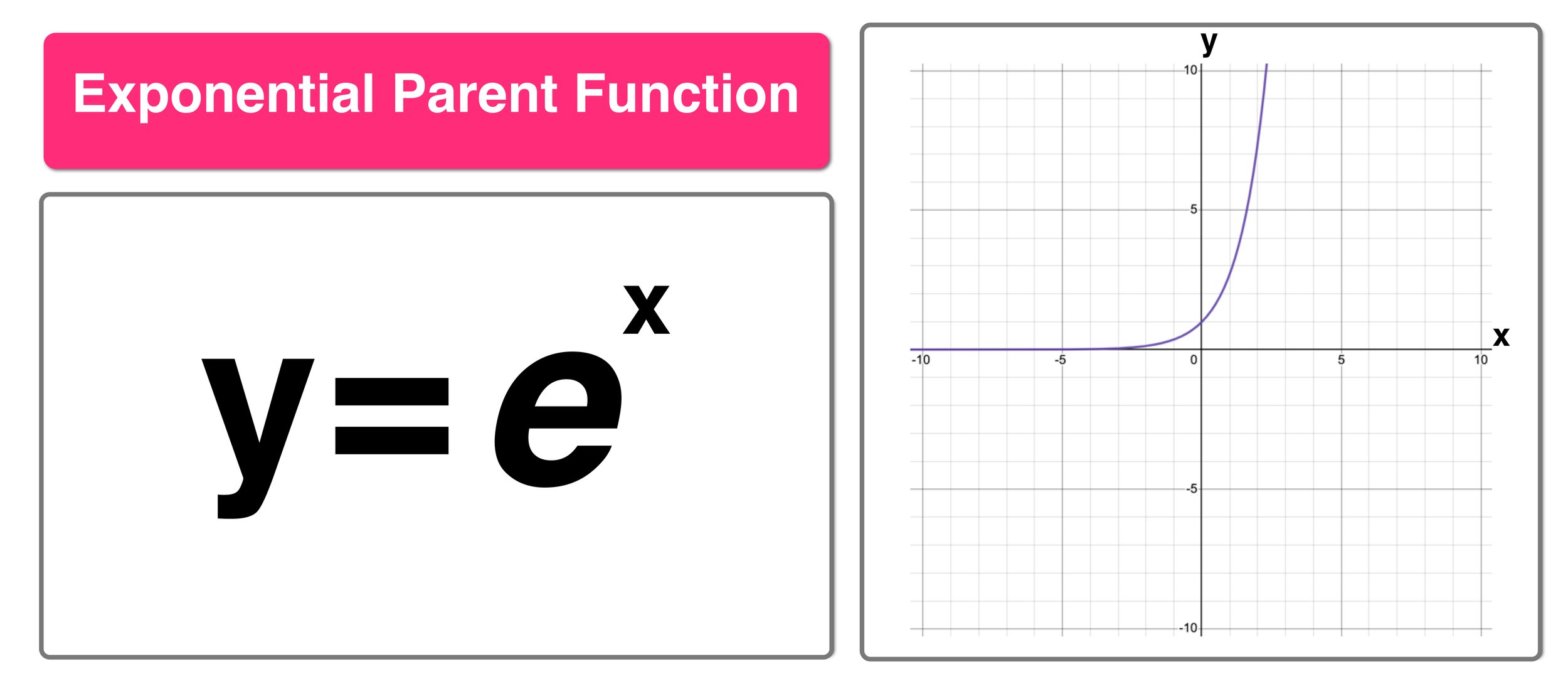
Understanding the Parent Function of Exponential Decay
Exponential decay is a fundamental concept in mathematics, describing a rapid decrease in quantity over time. This phenomenon is crucial in various fields, from physics and chemistry to finance and biology. At the heart of this concept lies the parent function of exponential decay, which serves as the foundation for understanding more complex decay models.
What is the Parent Function of Exponential Decay?
The parent function of exponential decay is represented by the equation:
y = a ⋅ bx, where 0 < b < 1. Here, ‘a’ is the initial value, and ‘b’ is the decay factor. This function illustrates how a quantity diminishes exponentially as ‘x’ increases.
📌 Note: The parent function is the simplest form of exponential decay, making it an essential starting point for analyzing more intricate decay scenarios.
Key Characteristics of the Parent Function
- Initial Value (a): Determines the starting point of the decay.
- Decay Factor (b): Controls the rate at which the quantity decreases. Since 0 < b < 1, the function always decreases.
- Asymptotic Behavior: As ‘x’ approaches infinity, ‘y’ approaches but never reaches zero.
| Parameter | Description |
|---|---|
| a | Initial value of the function |
| b | Decay factor (0 < b < 1) |
| x | Independent variable (time or other factor) |

Applications of Exponential Decay
Exponential decay is not just a theoretical concept; it has practical applications across various domains:
- Physics: Radioactive decay of elements.
- Finance: Depreciation of assets over time.
- Biology: Decay of medication in the bloodstream.
- Environmental Science: Reduction of pollutants in ecosystems.
How to Identify Exponential Decay in Real-World Scenarios
- Look for Rapid Initial Decline: Exponential decay starts with a sharp decrease.
- Consistent Half-Life: The time it takes for the quantity to halve remains constant.
- Asymptotic Approach to Zero: The quantity never reaches zero but gets infinitely close.
💡 Note: Understanding the parent function helps in modeling and predicting decay in real-world applications.
Summary Checklist
- Parent Function: y = a ⋅ bx (0 < b < 1)
- Key Parameters: Initial value (a), decay factor (b), and independent variable (x).
- Applications: Physics, finance, biology, and environmental science.
- Identification Tips: Rapid initial decline, consistent half-life, and asymptotic approach to zero.
What is the parent function of exponential decay?
+The parent function of exponential decay is y = a ⋅ bx, where 0 < b < 1. It represents the simplest form of exponential decrease.
Why is the decay factor always between 0 and 1?
+The decay factor (b) must be between 0 and 1 to ensure the function decreases exponentially over time.
How is exponential decay used in real life?
+Exponential decay is used in modeling radioactive decay, asset depreciation, medication decay, and pollutant reduction, among other applications.
In wrapping up, the parent function of exponential decay is a cornerstone in understanding how quantities diminish over time. By grasping its equation, characteristics, and applications, you can apply this knowledge to solve complex problems across various fields. Whether you’re a student, researcher, or professional, mastering this concept will enhance your analytical skills and problem-solving abilities.
Related Keywords: exponential decay function, decay factor, asymptotic behavior, radioactive decay, asset depreciation.