Mastering Pooled Variance Estimation for Better Data Analysis

<!DOCTYPE html>
In the world of data analysis, understanding variability is crucial for drawing accurate conclusions. Pooled variance estimation is a powerful technique that combines variance estimates from multiple groups, providing a more robust measure of variability. Whether you’re a data scientist, researcher, or statistician, mastering this method can significantly enhance your analytical skills. This guide will walk you through the essentials of pooled variance estimation, its applications, and how to implement it effectively. (pooled variance estimation, data analysis, statistical methods)
What is Pooled Variance Estimation?

Pooled variance estimation is a statistical method used to calculate a single, unified variance estimate from two or more independent groups. It is particularly useful when comparing group means or analyzing combined datasets. By pooling variances, you can achieve a more accurate and reliable measure of variability, especially when sample sizes are small or unequal. (variance estimation, statistical analysis, group comparison)
Why is Pooled Variance Important?
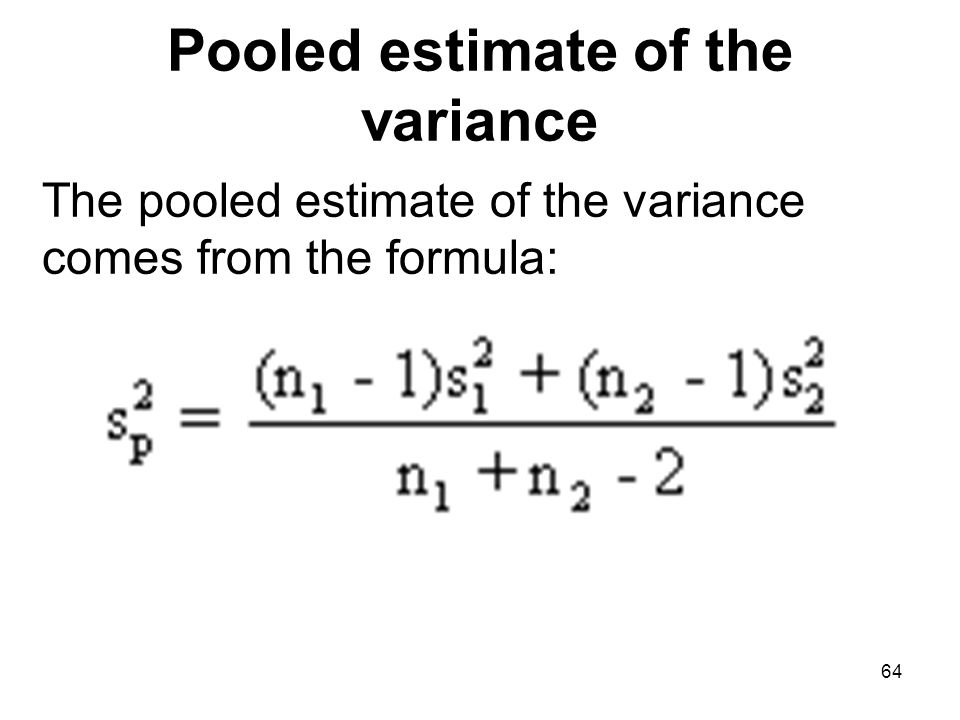
Pooled variance plays a critical role in various statistical tests, such as the two-sample t-test and ANOVA. It ensures that the variability across groups is adequately accounted for, leading to more precise inferences. Without proper variance pooling, your analysis might suffer from biased results or reduced statistical power. (statistical tests, two-sample t-test, ANOVA)
Steps to Calculate Pooled Variance

Calculating pooled variance involves a straightforward process. Here’s a step-by-step guide:
- Step 1: Gather Data – Collect the sample sizes, means, and variances for each group.
- Step 2: Calculate Pooled Variance – Use the formula:
$</td> <td>s_p^2 = \frac{\sum (n_i - 1) s_i^2}{\sum (n_i - 1)}</td> <td>$ 
where ( s_i^2 ) is the variance of the ( i^{th} ) group and ( n_i ) is its sample size. - Step 3: Interpret Results – Use the pooled variance in subsequent statistical analyses.
📊 Note: Ensure that the assumption of equal variances holds before applying pooled variance estimation.
Applications of Pooled Variance Estimation

Pooled variance estimation is widely used in:
- Medical Research – Comparing treatment effects across patient groups.
- Market Analysis – Analyzing consumer behavior in different demographics.
- Quality Control – Assessing variability in manufacturing processes.
Common Mistakes to Avoid

When working with pooled variance, avoid these pitfalls:
- Ignoring the assumption of equal variances.
- Using incorrect sample sizes in calculations.
- Misinterpreting results without considering group differences.
⚠️ Note: Always validate assumptions before proceeding with pooled variance estimation.
Checklist for Pooled Variance Estimation
Follow this checklist to ensure accurate pooled variance estimation:
- Verify the assumption of equal variances.
- Collect correct sample sizes and variances.
- Apply the pooled variance formula accurately.
- Interpret results in the context of your analysis.
Mastering pooled variance estimation is a valuable skill that can elevate your data analysis capabilities. By understanding its principles, applications, and potential pitfalls, you can make more informed decisions and draw reliable conclusions from your data. Whether you’re working in research, business, or academia, this technique is an essential tool in your statistical toolkit. (data analysis, statistical toolkit, informed decisions)
What is pooled variance estimation?
+Pooled variance estimation is a statistical method that combines variance estimates from multiple groups to provide a unified measure of variability.
When should I use pooled variance?
+Use pooled variance when comparing group means or analyzing combined datasets, especially when sample sizes are small or unequal.
What are the assumptions of pooled variance?
+The primary assumption is that the variances of the groups being compared are equal.



