Solve y = 2x + 3: Quick & Easy Steps

Struggling to solve the equation y = 2x + 3? Whether you're a student, a professional, or just brushing up on your algebra skills, this linear equation is a fundamental concept in mathematics. Understanding how to solve it not only helps in academics but also in real-world applications like finance, engineering, and data analysis. In this guide, we’ll walk you through the quick and easy steps to solve this equation, ensuring you grasp the process effortlessly. Let’s dive in and master this essential skill! (linear equations, algebra basics, solving equations)
Step-by-Step Guide to Solve y = 2x + 3
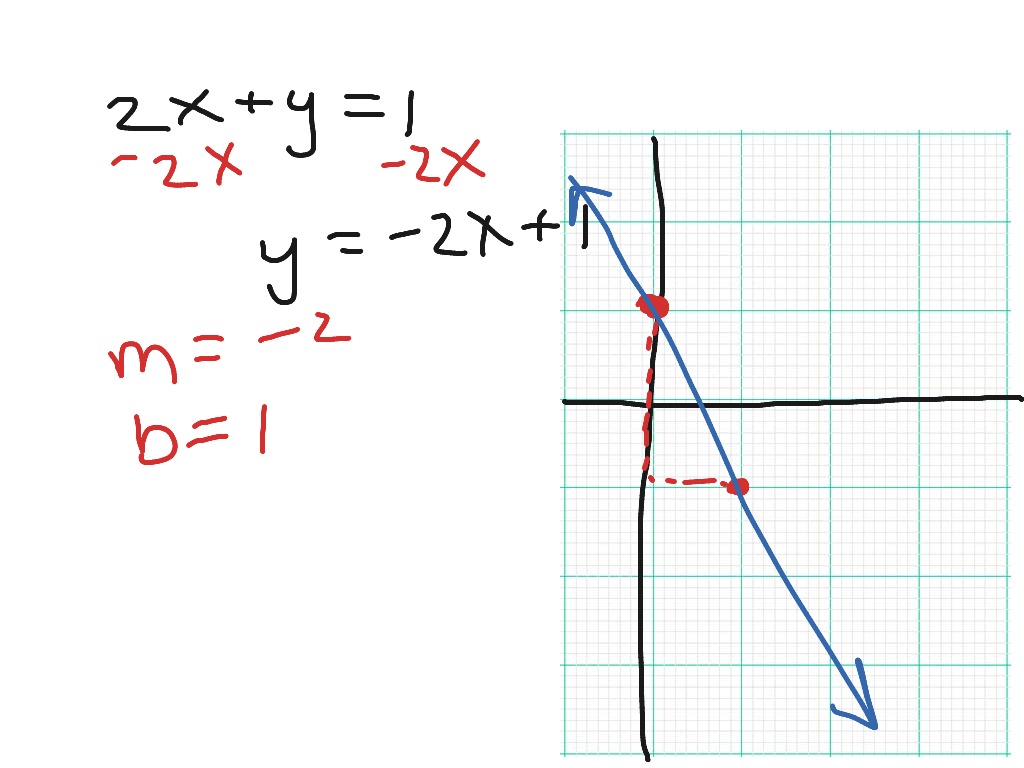
Solving the equation y = 2x + 3 involves understanding its components and applying basic algebraic principles. Follow these steps to solve it efficiently:
Step 1: Identify the Equation Type
The equation y = 2x + 3 is a linear equation in the slope-intercept form, y = mx + b, where:
- m is the slope (2 in this case)
- b is the y-intercept (3 in this case)
Recognizing this form is crucial for solving the equation. (slope-intercept form, linear equation)
Step 2: Isolate the Variable
To solve for x or y, isolate the variable on one side of the equation. For example, to solve for x when y is given:
- Subtract 3 from both sides: y - 3 = 2x
- Divide both sides by 2: x = (y - 3) / 2
📌 Note: Always perform the same operation on both sides to keep the equation balanced. (isolating variables, algebraic operations)
Step 3: Plug in Values (If Needed)
If you have a specific value for y, substitute it into the equation to find x. For instance, if y = 7:
x = (7 - 3) / 2 = 4 / 2 = 2
This process works similarly if you’re solving for y given a value for x. (substitution method, solving for x/y)
Practical Applications of Solving y = 2x + 3
Understanding how to solve y = 2x + 3 has numerous real-world applications. Here are a few examples:
| Field | Application |
|---|---|
| Finance | Calculating costs or profits based on variables. |
| Engineering | Designing systems with linear relationships. |
| Data Analysis | Plotting trends and predicting outcomes. |

Mastering this equation opens doors to more complex problem-solving. (real-world applications, linear relationships)
Solving the equation y = 2x + 3 is simpler than it seems when you break it down into manageable steps. By identifying the equation type, isolating variables, and substituting values, you can tackle this and similar problems with confidence. Practice makes perfect, so try applying these steps to different scenarios to reinforce your understanding. Happy solving! (algebra practice, problem-solving skills)
What is the slope-intercept form of a linear equation?
+
The slope-intercept form is y = mx + b, where m is the slope and b is the y-intercept.
How do I solve for y in the equation y = 2x + 3?
+
The equation is already solved for y. Simply substitute the value of x to find y.
Can this equation be used to graph a line?
+
Yes, the equation y = 2x + 3 can be graphed as a straight line with a slope of 2 and a y-intercept of 3.



